
In Part One, we looked at a very simple sequence of numbers that ‘orbited’ or homed-in on a certain value. Now we need to examine that value and look at the sheer magic of what it represents.
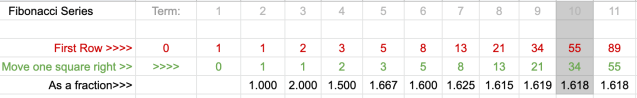
This new number was 1.618. It’s derivation is summarised in the diagram above, and described in the previous post. Simply: (red numbers) we add the two previous numbers to get the next. Next: (green numbers) we offset the first line of numbers one place to the right and, using a calculator to three decimal places, we treat the offset numbers of fractions, one number above the other. The third (black) line gives the calculator results, which stabilise at 1.618.
The ‘series’ that generates it – known as the Fibonacci series – came into existence at the time the world was abandoning the old and (by then) clumsy Roman notation (I, II, IV etc) and moving to the Arab-derived numerals that we use today.
The special number 1.618 is known by many names, such a the Golden Ratio and the Golden Mean. It is a number that shows us how we can divide something to protect its ‘wholeness’ in a harmonic way. By doing this, the divided figure will always exhibit pleasing proportions when placed next to (or within) the ‘parent’ figure. For example, Leonardo Da Vinci used it, extensively, in his most famous pictures.
But there are much deeper implications to this than something that looks or feels good, important though that is.
The materialist sees the world as having numbers by virtue of an ‘accident’ that they fit how we see and describe things. The mystic looks for the experience of ‘oneness’ with the processes that created the universe. You can’t find that experience unless you look for it. The universe owes us no debt of making it happen in our minds and hearts – the search must be ours… then the doors of perception will be opened.
Imagine that we have a strip of paper that we are going to divide by cutting with scissors. Let’s say the length of the initial strip is represented by the letter ‘A’. When we cut the strip we will have three values: the initial length (A); and the lengths of the two pieces we produce. We can name the two ‘child’ pieces (a) – the longest, and (b) – the shortest.
Under all circumstances, the original length (A) would be equal to the sum of the two children (a+b) . We can write this A=b+c, the most simple kind of ‘equation’ we could every want to see.
The miraculous Fibonacci number (given the name Phi in the 20th century) gives us the means to divide the original strip of paper such that the longer of the two child pieces bears the same relationship (ratio) to the original strip, as the larger child does to the smaller…
We can keep on doing this – cutting each successive larger portion – with smaller and smaller divisions of the original strip of paper. The whole ‘creation’ will be in harmonic proportions. This generation of smaller and smaller ‘harmonic’ children is called self-similarity.
Nature uses ‘Phi’ all the time. The recent science of Fractals shows how essential self-similar division is for nature to achieve its purposes. A tree is a fractal, for example, as are our lungs. Our blood vessels can carry oxygen to our cells because they follow fractal rules of becoming smaller and smaller within the finite space of our bodies. Only by using such structures can incredibly large processes fit into small spaces. The generation of Phi is not a fractal process, but it perfectly illustrates the marvel of the related fractal structures in nature.
Examples of this in nature include the petals of flowers, such as the sunflower, and the spirals of nautilus sea shells… But there are innumerable examples.
So, how would we actually work out the Phi-derived point of where to cut our twenty-unit strip of paper? We can arrange the self-similar formula so that we have a quadratic equation to solve, but where’s the fun in that!
Instead, we can look at the workings of the older graphical method carried out with the use of compass and straight edge. This brings home the inclusive and ‘connective’ nature of working by hand and is illustrated below:

The horizontal line A-B is the length of paper we wish to divide into the harmonic proportions given by the Fibonacci-derived Phi number 1.618. In this example, the length is 20 units.
To begin, we imagine we have turned the base line (A-B) into a square of four sides and select its right-hand vertical halfway point.
To shorten this, I have simply created point C at the correct half-value (10). The compass is placed on point C and set to the distance of C-B. We begin to draw an upward arc from B to the intersection with the hypotenuse A-C. We then set the compass to a base at the origin – A, and extend its pencil to the previous intersection with the hypotenuse. This time we draw downwards until the curve intersects with the original length A-B. The point of crossing is the length of the largest ‘child’ as above.
The length value, the golden ratio, gives us a new ‘longest child’ length of 12.36 units. We could cut at this point. The relationship of the larger child to the smaller is the same relationship as the original full length to the largest child.
This process could be repeated to infinity using the successive larger pieces. The entire family of larger pieces would inherit the divine proportions of the ‘mother’ length.
In the final post, next week, we will examine how the pentagram combines all the above properties into a single figure of dynamic value to mankind.
Other posts in this series:
One This is Two.
©Copyright Stephen Tanham
Stephen Tanham is a Director of the Silent Eye School of Consciousness, a not-for-profit teaching school of modern mysticism that helps people find a personal path to a deeper place within their internal and external lives.
The Silent Eye provides home-based, practical courses which are low-cost and personally supervised. The course materials and corresponding supervision are provided month by month without further commitment.
Steve’s personal blog, Sun in Gemini, is at stevetanham.wordpress.com.

Reblogged this on Stuart France.